Estimating Multiphase Hydraulic Properties at a Crude-Oil Spill Site

William Herkelrath, Hedeff Essaid, and Leslie Dillard

U.S. Geological Survey, Water Resources Division, Menlo Park, California

THREE-PHASE CAPILLARY PRESSURE CURVES:

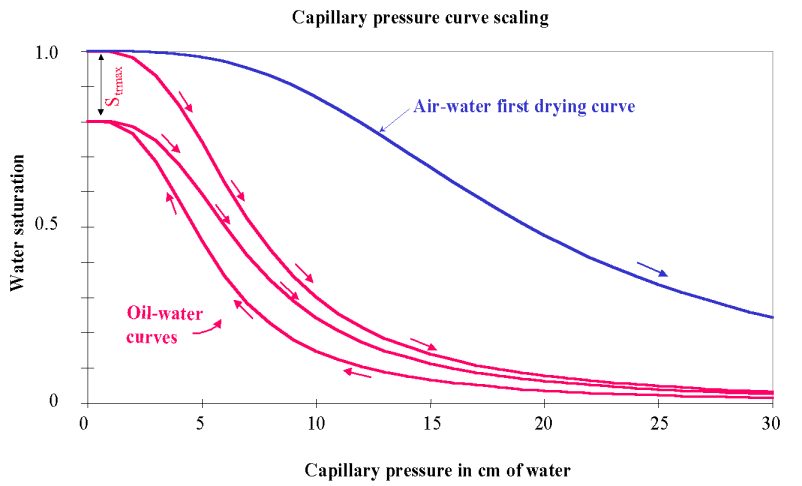
Oil-water and air-oil capillary pressure curves were scaled from the estimated air-water curve using the methods of Lenhard and Parker (1988), which were based on the concepts of Leverett and Lewis (1941). In this model, the sediment remains water wet, and the oil spreads on water, completely covering the air-water interface. For example, the oil-water capillary pressure, P_ow, was assumed to be a function of the water saturation, and calculated from the air-water curve by incorporating a scaling factor into the Van Genuchten equation

S_w = [1 + (b_owaP_ow)ⁿ]^-m,

where bow is the ratio of the air-water surface tension to the oil-water surface tension.

HYSTERESIS AND FLUID ENTRAPMENT

The hysteresis model we used was based on the work of Luckner, Van Genuchten, and Nielson (1989) and Kool and Parker (1987). For example, the main water wetting curve saturation was calculated from a modified Van Genuchten function:

S_w = [1-S_tr] [1 + (b_owa_wP_ow)ⁿ]^-m,

where a_w = 1.3 a, and S_tr = is the entrapped oil saturation. Oil entrapment was estimated using the model of Land (1968):

S_tr = S_rev / [1 - S_rev (1-1/S_trmax)] ,

where S_rev is the oil saturation at which reversal from drying to wetting occurred, and S_trmax is the maximum oil entrapment. Air entrapment was assumed to be zero. It was assumed that in the time scales of interest, entrapped air dissolved in the water or in the oil. An example hysteretic curve is shown below.

ESTIMATING RELATIVE PERMEABILITIES

At each grid point in the model aquifer, the water relative permeability, k_rw, was estimated using Mualem’s (1976) expression:

k_rw = (S_w)^1/2 {1 - [1-(S_w)^1/m]^m}²

where m = 1-1/n comes from the capillary pressure curve fit. The oil relative permeability, k_ro, was calculated using the modified formula suggested by Parker et al. (1987):

k_ro = (S_t - S_w)^1/2 {[1-(S_w)^1/m]^m - [1-(S_t)^1/m]^m}²,

where S_t is the total liquid saturation.

For comments and questions about this web page, email the Minnesota Webserver Team at:
bemidji@maildmnspl.cr.usgs.gov